
Analysis of Variance - Solved Problem 1
A researcher at an accounting firm wants to out whether the current ratio for three industries is about the same. Random samples of eight firms in industry A, six firms in industry B, and six firms in industry C are available. The ratios are as follows:
Industry A: 1.38, 1.55, 1.90, 2.00, 1.22, 2.11, 1.98, 1.61
Industry B: 2.33, 2.50, 2.79, 3.01, 1.99, 2.45
Industry C: 1.06, 1.37, 1.09, 1.65, 1.44, 1.11
Conduct the test at \(\alpha = 0.05\), and state your conclusion.
Solution: We are comparing the population mean ratio for three different industries. Our hypotheses are. Let's call![]() ,
,![]() ,
, ![]() , to those mean ratios, our hypotheses are
, to those mean ratios, our hypotheses are
![]()
We use ANOVA (analysis of variance) to test this hypothesis. One of the assumptions for ANOVA is that the population variances are the same. We’ll use an F-test to compare all thee pairs of variances, obtaining that
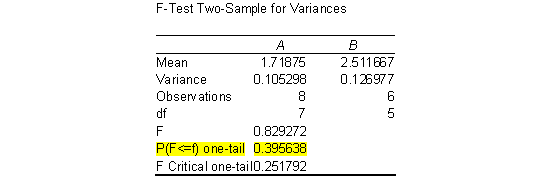
|
F-Test Two-Sample for Variances | ||
|
B |
C | |
| Mean |
2.511666667 |
1.286667 |
| Variance |
0.126976667 |
0.056747 |
| Observations |
6 |
6 |
| df |
5 |
5 |
| F |
2.237605733 |
|
|
P(F<=f) one-tail |
0.198705266 |
|
|
F Critical one-tail |
5.050338814 |
|

All the p-values are greater than 0.05, the level of significance, and therefore none of them is significant, which means that we can consider all the population variances to be equal. Now we can safely apply the ANOVA procedure. Using EXCEL we get
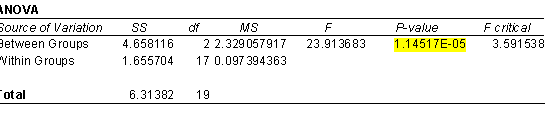
Here we observe that the p-value is significant (because it's less than 0.05) which means that we reject the null hypothesis, and we accept the alternative. Therefore, the current ratio for three industries cannot be considered the same, for the given level of significance.

Related Content


